The term 'oblique' in relation to map projections means that ? [ Formation assignment ]
Question 161-1 : The axis of the cylinder or cone is neither parallel to or perpendicular to the earth's axis of rotation the axis of the cylinder or cone is parallel to the earth's axis of rotation the axis of the cylinder or cone is perpendicular to the earth's axis of rotation the axis of the cylinder or cone is parallel and perpendicular to the earth's axis of rotation
 The axis of the cylinder or cone is neither parallel to or perpendicular to the earth's axis of rotation.
The axis of the cylinder or cone is neither parallel to or perpendicular to the earth's axis of rotation. A day at a place as measured in local mean time starts ?
Question 161-2 : When the mean sun transits the anti meridian of the place in question when the real sun transits the meridian of the place in question when the real sun transits the greenwich meridian when the mean sun transits the 180e/w meridian
 When the mean sun transits the anti meridian of the place in question.
When the mean sun transits the anti meridian of the place in question. Standard time is ?
Question 161-3 : The time enforced by the legal authority to be used in a country or an area the time which is accepted and used as a standard for the whole world the time most frequently used for air navigation the time used at a particular meridian
 The time enforced by the legal authority to be used in a country or an area.
The time enforced by the legal authority to be used in a country or an area. Daylight saving time summer time is .1 used to extend the sunlight period in ?
Question 161-4 : 1 2 3 1 3 1 2 2
 1, 2, 3.
1, 2, 3. The countries having a standard time slow on utc ?
Question 161-5 : Will generally be located at western longitudes will often have an earlier standard date than the utc date will often experience sunrise earlier than the sunrise occurs at the greenwich meridian will generally be located at eastern longitudes
 Will generally be located at western longitudes.
Will generally be located at western longitudes. The international date line is located ?
Question 161-6 : At the 180° e/w meridian or in the vicinity of this meridian at the apparent suns anti meridian at the greenwich meridian at all latitudes on the 180° e/w meridian
 At the 180° e/w meridian, or in the vicinity of this meridian.
At the 180° e/w meridian, or in the vicinity of this meridian. Consider the following statements on sunset ?
Question 161-7 : Sunset is the time when the observer at sea level sees the last part of the sun disappear below the horizon for positions at the same longitude sunset will occur simultaneously at all latitudes at sunset the centre of the sun is at the observers horizon night flying regulations start at the time of sunset
 Sunset is the time when the observer at sea level sees the last part of the sun disappear below the horizon.
Sunset is the time when the observer at sea level sees the last part of the sun disappear below the horizon. Atmospheric refraction ?
Question 161-8 : Causes the sunrise to occur earlier and the sunset to occur later causes the sunrise and the sunset to occur earlier causes the sunrise and the sunset to occur later causes the sunrise to occur later and the sunset to occur earlier
 Causes the sunrise to occur earlier and the sunset to occur later.
Causes the sunrise to occur earlier and the sunset to occur later. Consider the following statements on sunrise and sunset ?
Question 161-9 : At equator sunrise and sunset occur at quite regular times throughout the year in may sunrise occurs later at 45°n than at 45°s in november sunset occurs earlier at 45°s than at 45°n in july the period of sunlight is longer at 15°s than at 15°n
 At equator sunrise and sunset occur at quite regular times throughout the year.
At equator sunrise and sunset occur at quite regular times throughout the year. 'true north' is ?
Question 161-10 : The direction along any meridian toward the true north pole the direction along the meridian toward the north pole when on the northern hemisphere and toward the south pole when on the southern hemisphere in any direction out from the true north pole the direction along a meridian
 The direction along any meridian toward the true north pole.
The direction along any meridian toward the true north pole. In international aviation the following units shall be used for horizontal ?
Question 161-11 : Metres kilometres and nautical miles kilometres statute miles and nautical miles metres statute miles and nautical miles kilometres feet and nautical miles
 Metres, kilometres and nautical miles.
Metres, kilometres and nautical miles. When dealing with heights and altitudes in international aviation we use the ?
Question 161-12 : Metre and feet feet kilometre and decimals of nautical mile feet and yard statute mile and feet
 Metre and feet.
Metre and feet. 'kilometre' is defined as ?
Question 161-13 : A 1/10000 part of the meridian length from equator to the pole 0 621 statute mile 0 454 nautical mile the mean length of a 1/40000 part of the equator
 A 1/10000 part of the meridian length from equator to the pole.
A 1/10000 part of the meridian length from equator to the pole. How long is 25 kilometres at 60°n ?
Question 161-14 : 13 5 nautical mile 40 2 statute miles 46 3 nautical mile 27 0 nautical mile
 13.5 nautical mile.
13.5 nautical mile. Position a 50°00 0'n 138°30 0'w st a = utc 9 h .position b 50°00 0'n ?
Question 161-15 : 09 00 st b 05/02 05 00 st b 03/02 05 00 st b 05/02 12 00 st b 04/02
 09:00 st(b) 05/02.
09:00 st(b) 05/02. Estimated time of departure a 15°15 0'n 072°06 0'w on 12 march is 01 00 st st ?
Question 161-16 : 09h35m and 02h42m 04h35m and 02h42m 07h35m and 01h42m 12h35m and 04h32m
 09h35m and 02h42m.
09h35m and 02h42m. What is meant by the term 'polar circle' ?
Question 161-17 : It is the parallel at the lowest latitude at which an observer can see the sun for 24 hours above the horizon it is the parallel at the highest latitude at which an observer can see the sun for 12 hours above the horizon it is the parallel at the lowest latitude at which an observer can see the sun for 12 hours above the horizon it is the parallel at the highest latitude at which an observer can see the sun for 24 hours above the horizon
 It is the parallel at the lowest latitude at which an observer can see the sun for 24 hours above the horizon.
It is the parallel at the lowest latitude at which an observer can see the sun for 24 hours above the horizon. Grivation is 56w when ?
Question 161-18 : Gh is 103° and mh is 159° gh is 156° and mh is 103° grid convergence is 46w and variation is 10e grid convergence is 58w and deviation is 2e
 Gh is 103° and mh is 159°.
Gh is 103° and mh is 159°. The term 'ellipsoid' may be used to describe ?
Question 161-19 : The shape of the earth the movement of the earth around the sun the shape of the ecliptic a great circle on the celestial sphere
 The shape of the earth.
The shape of the earth. By 'ecliptic' is meant ?
Question 161-20 : The apparent yearly path of the sun around the earth the apparent yearly path of the earth around the sun the path of the moon around the sun the path of the moon around the earth
 The apparent yearly path of the sun around the earth.
The apparent yearly path of the sun around the earth. The duration of civil twilight is the time ?
Question 161-21 : Between sunset and when the centre of the sun is 6° below the celestial horizon agreed by the international aeronautical authorities which is 12 minutes needed by the sun to move from the apparent height of 0° to the apparent height of 6° between sunset and when the centre of the sun is 12° below the celestial horizon
 Between sunset and when the centre of the sun is 6° below the celestial horizon.
Between sunset and when the centre of the sun is 6° below the celestial horizon. An aircraft is over position ho 55°30'n 060°15'w where yyr vor 53°30'n ?
Question 161-22 : 028° 208° 031° 332°
 028°.
028°. An aircraft in the northern hemisphere is making an accurate rate one turn to ?
Question 161-23 : More than 225° 225° less than 225° more or less than 225° depending on the pendulous suspension used
 More than 225°.
More than 225°. When accelerating on a westerly heading in the northern hemisphere the compass ?
Question 161-24 : Anti clockwise giving an apparent turn towards the north anti clockwise giving an apparent turn towards the south clockwise giving an apparent turn towards the north clockwise giving an apparent turn towards the south
 Anti-clockwise giving an apparent turn towards the north.
Anti-clockwise giving an apparent turn towards the north. A ground feature appears 30° to the left of the centre line of the crt of an ?
Question 161-25 : 160° 220° 130° 310°
 160°.
160°. When decelerating on a westerly heading in the northern hemisphere the compass ?
Question 161-26 : Clockwise giving an apparent turn toward the south anti clockwise giving an apparent turn towards the south clockwise giving an apparent turn towards the north anti clockwise giving an apparent turn towards the north
 Clockwise giving an apparent turn toward the south.
Clockwise giving an apparent turn toward the south. When turning right from 330° c to 040° c in the northern hemisphere the ?
Question 161-27 : Under indicate the turn and liquid swirl will increase the effect over indicate the turn and liquid swirl will decrease the effect under indicate the turn and liquid swirl will decrease the effect over indicate the turn and liquid swirl will increase the effect
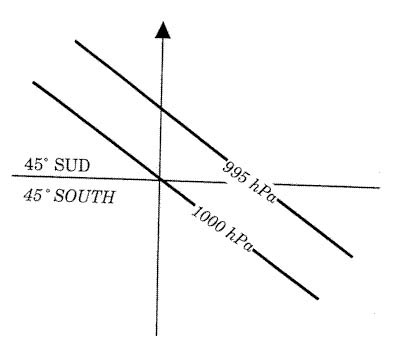 Under-indicate the turn and liquid swirl will increase the effect.
Under-indicate the turn and liquid swirl will increase the effect. When accelerating on an easterly heading in the northern hemisphere the compass ?
Question 161-28 : Clockwise giving an apparent turn toward the north clockwise giving an apparent turn toward the south anti clockwise giving an apparent turn toward the north anti clockwise giving an apparent turn toward the south
 Clockwise giving an apparent turn toward the north.
Clockwise giving an apparent turn toward the north. A direct reading compass should be swung when ?
Question 161-29 : There is a large and permanent change in magnetic latitude there is a large change in magnetic longitude the aircraft is stored for a long period and is frequently moved the aircraft has made more than a stated number of landings
 There is a large, and permanent, change in magnetic latitude.
There is a large, and permanent, change in magnetic latitude. The direct reading magnetic compass is made aperiodic dead beat by ?
Question 161-30 : Keeping the magnetic assembly mass close to the compass point and by using damping wires pendulous suspension of the magnetic assembly using long magnets using the lowest acceptable viscosity compass liquid
 Keeping the magnetic assembly mass close to the compass point and by using damping wires.
Keeping the magnetic assembly mass close to the compass point and by using damping wires. Given .true track is 348° drift 17° left variation 32°w deviation 4°e .what ?
Question 161-31 : 033° 007° 359° 337°
 033°.
033°. Which of the following statements is correct concerning the effect of turning ?
Question 161-32 : Turning errors are greatest on north/south headings and are greatest at high latitudes turning errors are greatest on east/west headings and are least at high latitudes turning errors are greatest on north/south headings and are least at high latitudes turning errors are greatest on east/west headings and are greatest at high latitudes
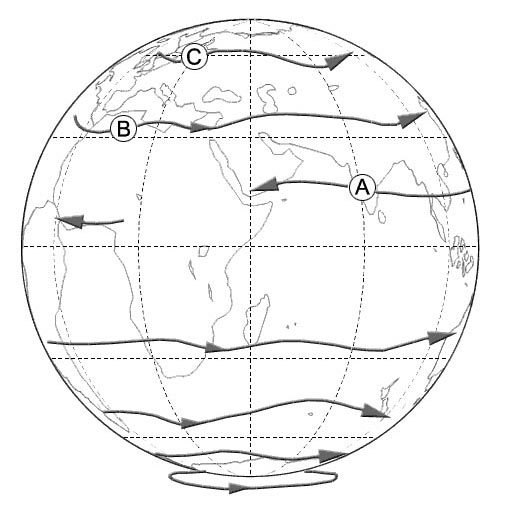 Turning errors are greatest on north/south headings, and are greatest at high latitudes
Turning errors are greatest on north/south headings, and are greatest at high latitudes Which of the following is an occasion for carrying out a compass swing on a ?
Question 161-33 : After an aircraft has passed through a severe electrical storm or has been struck by lightning before an aircraft goes on any flight that involves a large change of magnetic latitude after any of the aircraft radio equipment has been changed due to unserviceability whenever an aircraft carries a large freight load regardless of its content
 After an aircraft has passed through a severe electrical storm, or has been struck by lightning.
After an aircraft has passed through a severe electrical storm, or has been struck by lightning. When is the magnetic compass most effective ?
Question 161-34 : About midway between the magnetic poles in the region of the magnetic south pole in the region of the magnetic north pole on the geographic equator
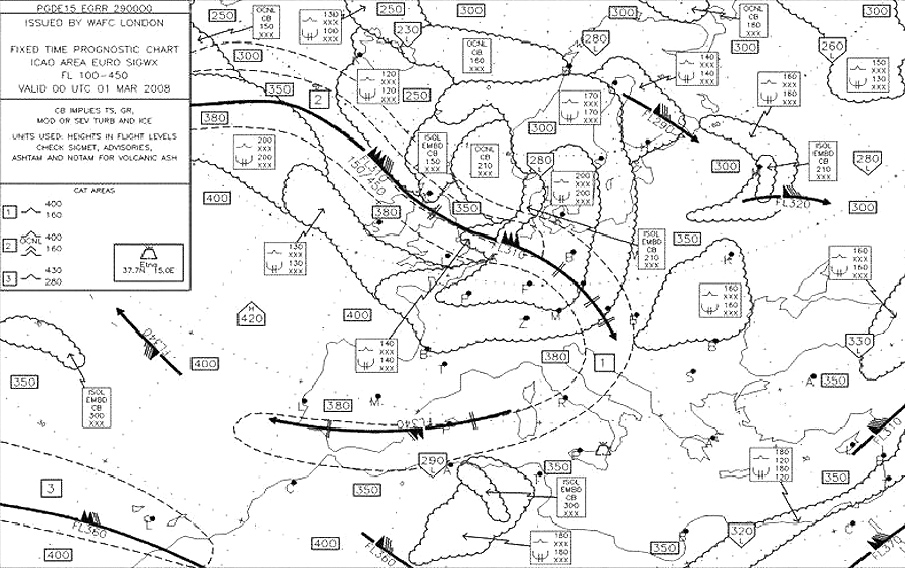 About midway between the magnetic poles.
About midway between the magnetic poles. When an aircraft on a westerly heading on the northern hemisphere accelerates ?
Question 161-35 : Indicate a turn towards the north lag behind the turning rate of the aircraft indicate a turn towards the south to turn faster than the actual turning rate of the aircraft
 Indicate a turn towards the north.
Indicate a turn towards the north. Given .true track 180°.drift 8°r.compass heading 195°.deviation 2° ?
Question 161-36 : 21°w 25°w 5°w 9°w
 21°w.
21°w. Compass deviation is defined as the angle between ?
Question 161-37 : Magnetic north and compass north true north and magnetic north true north and compass north the horizontal and the total intensity of the earth's magnetic field
 Magnetic north and compass north.
Magnetic north and compass north. Given .true course 300° drift 8°r variation 10°w deviation 4° .calculate ?
Question 161-38 : 306° 322° 294° 278°
 306°.
306°. Given .true track 352° variation 11° w deviation is 5° drift 10°r ?
Question 161-39 : 358° 346° 018° 025°
 358°.
358°. Given .true track 070°.variation 30°w.deviation +1°.drift 10°r .calculate ?
Question 161-40 : 089° 091° 100° 101°
 089°.
089°. ~
Exclusive rights reserved. Reproduction prohibited under penalty of prosecution.
6399 Free Training Exam
